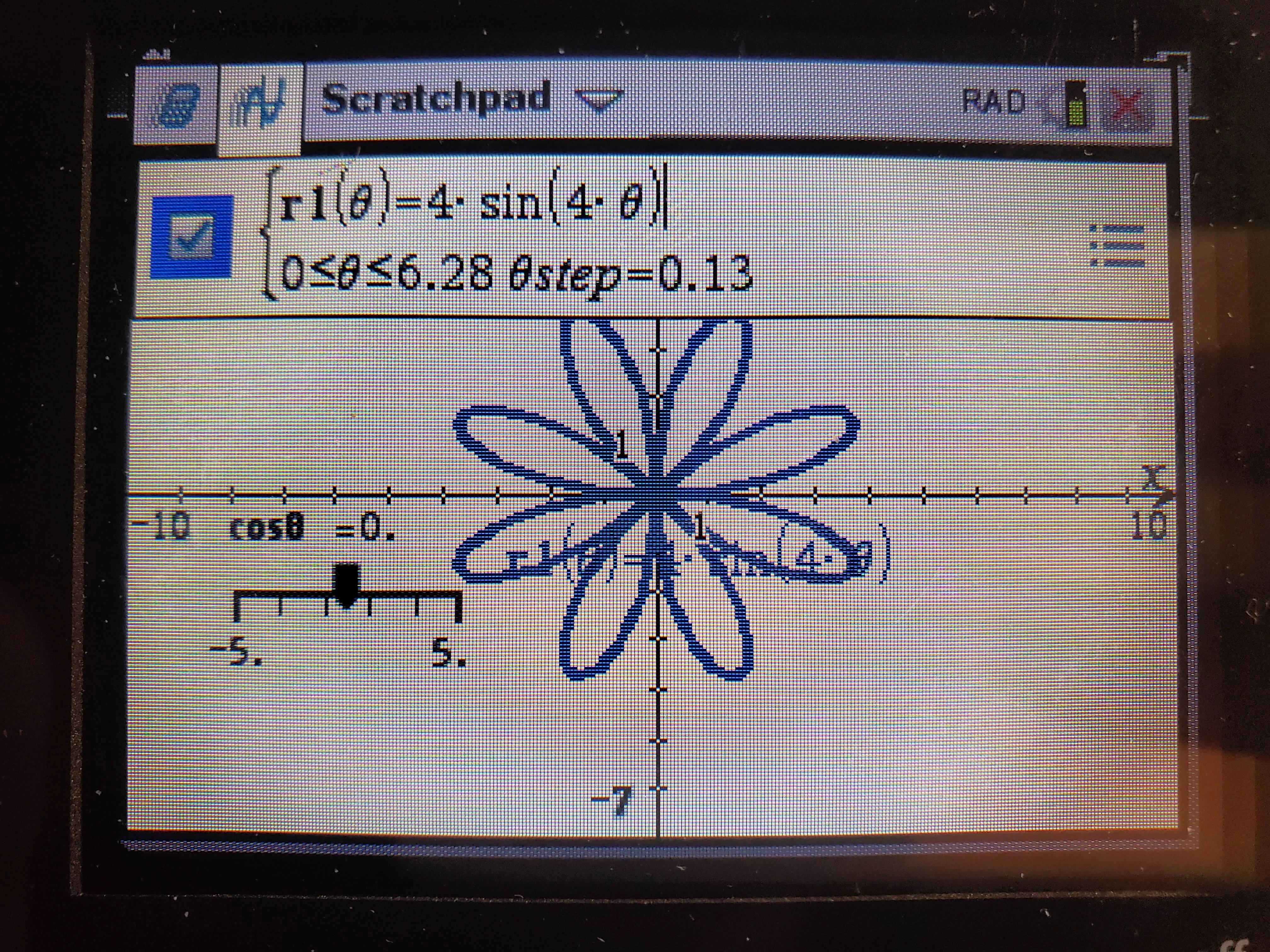
Boys, set your calculator to polar mode, and draw a graph of $r=4sin(3θ)$, then set the first interval of [0,π] and the second one: [0, 2π] [0,π] [0,2π] They looks exactly the same, isn’t it? And if we take of the integral of these two to find the area, for the graph with interval [0,π], area = 4π, but for the graph with [0,2π] the area is 8π, thus, which one is the “true” one we want to find?